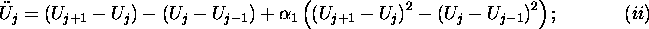
Stanislaw Ulam can be thought of as the father of cellular automata. He began investigating discrete cellular automata in 1950 [1]. In 1955, he co-authored the famous paper, [2], with Fermi and Pasta, which has been the starting point of many works in nonlinear waves and solitons.
In an introduction to [2], written by Ulam in 1965, he remarks that
``Fermi expressed often a belief that future fundamental theories in physics may involve nonlinear operators and equations, and that it would be useful to attempt practice in the mathematics needed for the understanding of nonlinear systems. The plan was then to start with the possibly simplest such physical model and to study the results of the calculation of its long-time behavior."[3]
In their 1955 paper, Fermi, Pasta, and Ulam examined nonlinear models for particles attached horizontally by springs where the particles can move vertically. We refer to this model as the FPU Model. Fermi, Pasta, and Ulam considered two algorithms for the resulting wave motion. The first corresponds to a quadratic nonlinearity:
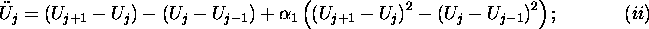
the second corresponds to a cubic nonlinearity
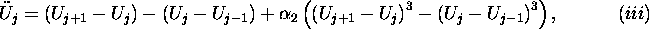
where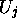 
represents the vertical position of the j
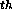particle
and
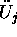is the second derivative of the j
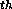particle's position with
respect to time. Fermi, Pasta, and Ulam point out that for each of these schemes
``(t)he corresponding partial differential equation...is the usual wave equation
plus nonlinear terms of a complicatied nature."
In section 2 of our paper, we determine the precise form of the nonlinear terms of the hyperbolic partial differential equations that correspond to schemes (ii) and (iii). Section 3 of our paper discusses the accuracy and stability of cellular automata simulations for the FPU model with an eye to finding reasonable parameter values to use in running the nonlinear wave schemes. In particluar we address the role of characteristics in determining the Courant-Frederichs-Lewy stability condition.
In the past it has been customary to study only cellular automata which have a finite number of discrete state values. In Section 4 of our paper, we show how it is natural to extend the idea of cellular automata to rules which allow continuous-valued state variables. We describe how specific continuous-valued cellular automata simulations for the linear and nonlinear waves were finally arrived at, resulting in the shareware program CAPOW [4].
Section 5 shows some illustrations generated by CAPOW and discusses their meaning. In particular, we examine the behavior of the quadratic and cubic nonlinear waves, study the ways in which instability can set in for nonlinear waves, and confirm an observation of [2] that a nonlinear wave seeded with a sine wave will eventually cycle back to a close (but not exact) approximation of the initial sine wave seed. Further we examine a way in which unstable nonlinear wave simulations can be made to behave like discrete-valued cellular automata. This effect occurs when the possible cell values are clamped to lie in a bounded range. A clamped unstable CA rule bounces among the minimum and maximum values and a few in-between values, producing what is effectively a discrete-valued CA. To coin a word, we might refer to clamped unstable continuous-valued cellular automata with a finite range of values as ``pseudodiscrete CAs". Our experiments indicate that pseudodiscrete CAs can be of Wolfram class 1, 2, 3, or 4, i.e. single-valued, periodic, chaotic, or complex. [5]