

Next: Accuracy and Stability
Up: Continuous Valued Cellular
Previous: Introduction
We begin by describing the nonlinear string in the FPU model in more detail.
When the string is at rest it lies along the
x-axis. When the string moves it only moves in the y
direction. The string is modeled by considering a series of particles along
the x-axis with fixed x coordinates that are separated by a distance,
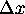 (so it follows that the mass of each particle is
(so it follows that the mass of each particle is  , where
, where
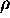 is the constant density of the string). Each particle's motion in the
y direction is modeled by considering the effect of springs connecting each
particle to its two neighbors. In Rheology, long polymeric molecules
are often modeled by considering these ``bead-spring" type models, where each
bead/particle represents 10-30 monomer units. When the springs are
assumed to follow Hooke's law, these macromolecular models are called Rouse
chains; when the springs are not Hookean, they are called Rouse-Zimm
chains. [6]
is the constant density of the string). Each particle's motion in the
y direction is modeled by considering the effect of springs connecting each
particle to its two neighbors. In Rheology, long polymeric molecules
are often modeled by considering these ``bead-spring" type models, where each
bead/particle represents 10-30 monomer units. When the springs are
assumed to follow Hooke's law, these macromolecular models are called Rouse
chains; when the springs are not Hookean, they are called Rouse-Zimm
chains. [6]
Let 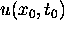 represent the vertical displacement (i.e., the value of y) at time
represent the vertical displacement (i.e., the value of y) at time
 of the particle whose horizontal location is
of the particle whose horizontal location is 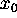 , and let
, and let 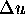 represent
the difference in the vertical displacement between two neighboring particles. We
can express the distance,
represent
the difference in the vertical displacement between two neighboring particles. We
can express the distance,
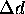 , between two neighboring particles as
, between two neighboring particles as 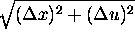 . The
magnitude of the force for most springs will usually be some function
G of this distance (divided by
. The
magnitude of the force for most springs will usually be some function
G of this distance (divided by
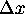 so that the force is normalized), i.e.,
so that the force is normalized), i.e.,

Since it is assumed that the particles do not move in the x
direction, we are only concerned with the vertical component of the spring force.
The absolute value of the force's vertical component is given by

which we can express strictly as a function of 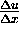 :
:

If the spring is linear (i.e., follows Hooke's law) and has an equilibrium length of
z=0, as is the case for Rouse chains, then
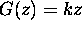 , where
k is the positive-valued spring constant, and therefore
, where
k is the positive-valued spring constant, and therefore
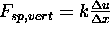 . For linear springs with
nonzero equilibrium lengths or nonlinear springs where
. For linear springs with
nonzero equilibrium lengths or nonlinear springs where
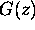 is an analytic function near z=1, we can express
is an analytic function near z=1, we can express
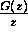 as a series of even powers of
as a series of even powers of 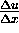 when
when 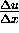 is small, which leads to the following expression for
is small, which leads to the following expression for  :
:
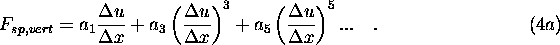
If the spring force is an analytic function of 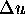 instead of an analytic function of distance, we may also have even powers in the
series:
instead of an analytic function of distance, we may also have even powers in the
series:
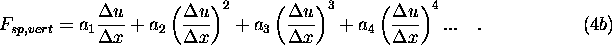
Now we consider the effect of the forces on a generic particle,
located at
 . If we define
. If we define 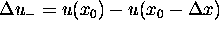 and
and 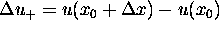 then we can express Newton's law by using eq (4b) to describe
the effect of each of the two springs acting on the particle:
then we can express Newton's law by using eq (4b) to describe
the effect of each of the two springs acting on the particle:

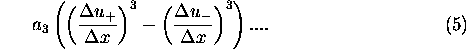
As 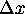 approaches 0 in eq (5), we obtain the nonlinear partial differential
equation corresponding to the FPU model:
approaches 0 in eq (5), we obtain the nonlinear partial differential
equation corresponding to the FPU model:
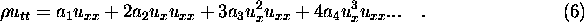
We consider some special cases of our model. If the springs follow Hooke's law and
have an equilibrium length of 0, then the nonlinear terms disappear and we are left
with the standard linear wave equation

where 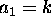 , the spring constant. If
, the spring constant. If  stays small in the nonlinear model
we can ignore the effects of higher order terms. This leads to the quadratic FPU
equation
stays small in the nonlinear model
we can ignore the effects of higher order terms. This leads to the quadratic FPU
equation

which FPU's scheme (ii) simulated.
When the spring force is strictly a function of distance, we work with eq (4a)
instead of eq (4b), so 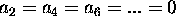 . For this common
case, we obtain the cubic FPU equation when
. For this common
case, we obtain the cubic FPU equation when 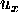 stays small
stays small

which FPU's scheme (iii) simulated.
Eqs (7)-(9) will be the three equations of interest in this paper.


Next: Accuracy and Stability
Up: Continuous Valued Cellular
Previous: Introduction
Rudy Rucker
Sat May 9 20:21:15 MET DST 1998
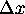 (so it follows that the mass of each particle is
(so it follows that the mass of each particle is  , where
, where
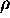 is the constant density of the string). Each particle's motion in the
y direction is modeled by considering the effect of springs connecting each
particle to its two neighbors. In Rheology, long polymeric molecules
are often modeled by considering these ``bead-spring" type models, where each
bead/particle represents 10-30 monomer units. When the springs are
assumed to follow Hooke's law, these macromolecular models are called Rouse
chains; when the springs are not Hookean, they are called Rouse-Zimm
chains. [6]
is the constant density of the string). Each particle's motion in the
y direction is modeled by considering the effect of springs connecting each
particle to its two neighbors. In Rheology, long polymeric molecules
are often modeled by considering these ``bead-spring" type models, where each
bead/particle represents 10-30 monomer units. When the springs are
assumed to follow Hooke's law, these macromolecular models are called Rouse
chains; when the springs are not Hookean, they are called Rouse-Zimm
chains. [6]
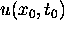 represent the vertical displacement (i.e., the value of y) at time
represent the vertical displacement (i.e., the value of y) at time
 of the particle whose horizontal location is
of the particle whose horizontal location is 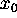 , and let
, and let 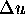 represent
the difference in the vertical displacement between two neighboring particles. We
can express the distance,
represent
the difference in the vertical displacement between two neighboring particles. We
can express the distance,
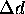 , between two neighboring particles as
, between two neighboring particles as 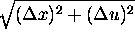 . The
magnitude of the force for most springs will usually be some function
G of this distance (divided by
. The
magnitude of the force for most springs will usually be some function
G of this distance (divided by
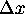 so that the force is normalized), i.e.,
so that the force is normalized), i.e.,


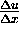 :
:

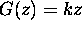 , where
k is the positive-valued spring constant, and therefore
, where
k is the positive-valued spring constant, and therefore
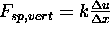 . For linear springs with
nonzero equilibrium lengths or nonlinear springs where
. For linear springs with
nonzero equilibrium lengths or nonlinear springs where
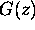 is an analytic function near z=1, we can express
is an analytic function near z=1, we can express
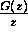 as a series of even powers of
as a series of even powers of 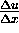 when
when 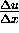 is small, which leads to the following expression for
is small, which leads to the following expression for  :
:
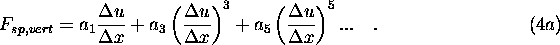
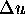 instead of an analytic function of distance, we may also have even powers in the
series:
instead of an analytic function of distance, we may also have even powers in the
series:
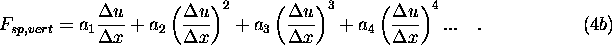
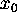 . If we define
. If we define 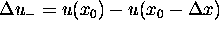 and
and 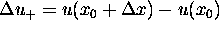 then we can express Newton's law by using eq (4b) to describe
the effect of each of the two springs acting on the particle:
then we can express Newton's law by using eq (4b) to describe
the effect of each of the two springs acting on the particle:

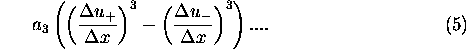
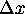 approaches 0 in eq (5), we obtain the nonlinear partial differential
equation corresponding to the FPU model:
approaches 0 in eq (5), we obtain the nonlinear partial differential
equation corresponding to the FPU model:
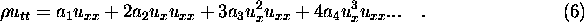

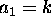 , the spring constant. If
, the spring constant. If  stays small in the nonlinear model
we can ignore the effects of higher order terms. This leads to the quadratic FPU
equation
stays small in the nonlinear model
we can ignore the effects of higher order terms. This leads to the quadratic FPU
equation

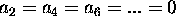 . For this common
case, we obtain the cubic FPU equation when
. For this common
case, we obtain the cubic FPU equation when 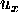 stays small
stays small
